
Formative assessment, developmental stages and starting the year well
The goal of formative assessment should always be to find out what each student NEEDS next, rather than focusing

I am often asked what makes a perfect mathematics lesson. This is a difficult question to answer, as I’m not sure that there is one best lesson. It really depends on what you want to achieve, as the purpose of a mathematics lesson determines its structure. I find that kids adapt well to different types of lessons, as long as they know why they are doing them.
Start by working out what you want to achieve in your mathematics lesson today:
Next, it is important to set the scene for students and explain what we will be doing today.
Finally, set success criteria in line with your purpose:
Have a great time and remember to let me know how it goes.
Tierney
Want more?

The goal of formative assessment should always be to find out what each student NEEDS next, rather than focusing
Recently I’ve been pondering findings from a major report into Australian schooling that kids who are struggling in maths by
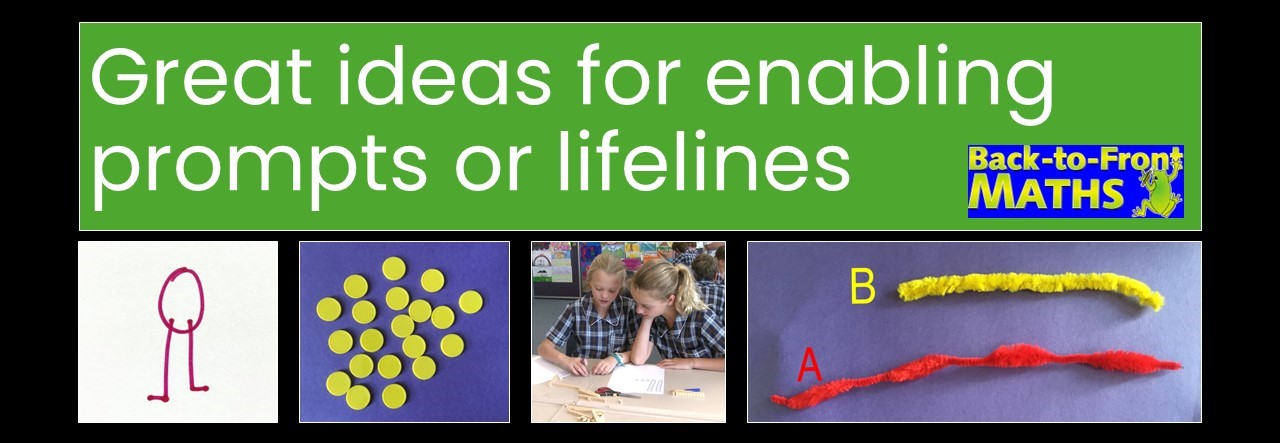
Enabling prompts or life lines are a fantastic way of helping students who are stuck to get started. They do not reduce

Pie charts are an awesome way of linking statistics, fractions and angles, however they can often be difficult for students

While it may sound counter-intuitive, the easiest way to learn to tell the time is to remove the minute hand
Hundreds charts are great for connecting tens and ones. Why not turn one into a jigsaw puzzle to use in

KENNEDY PRESS PTY LTD
FOR ALL ENQUIRIES, ORDERS AND TO ARRANGE PD:
© COPYRIGHT 2023 KENNEDY PRESS PTY LTD ALL RIGHTS RESERVED TERMS & CONDITIONS